A New Facet of Cubism:
‘The Fourth Dimension’ and ‘Non-Euclidean Geometry’ Reinterpreted

By Linda Dalrymple Henderson

[Page 411:]

Historians of modern art have frequently attempted to relate developments in early twentieth-century art to those of contemporary science, and a number of authors in the last thirty years have assumed or alluded to the positive effect upon Cubism of Einstein’s Special Theory of Relativity of 1905 and Minkowski’s formulation of the space-time continuum in 1908. But since no reference to Einstein or Minkowski ever occurs in Cubist literature, proponents of the connection of Cubism with relativity have relied solely on the use of the terms “the fourth dimension” and “non-Euclidean geometry” by Apollinaire and by Gleizes and Metzinger. The following passage from Paul Laporte’s article “Cubism and Science,” of 1949, is perhaps the clearest statement of this approach:

It may very well be argued ... that the introduction of non-Euclidean geometry into physics on the one hand, and the breaking away from occidental perspective on the other hand, are correlative movements in the evolution of the western mind. Furthermore, the new pictorial idiom created by cubism is most satisfactorily explained by applying to it the concept of the space-time continuum. That this explanation is legitimate is at least indicated by Apollinaire’s references to non-Euclidean geometry and the fourth dimension.

. . . . . . . .

The integration of non-Euclidean geometry with the fourth dimension is a constituent factor in contemporary physics. This happened in physics at exactly the same time as the change to cubism happened in painting (Einstein, Special Theory of Relativity, 1905; Minkowski, 1908; Picasso’s first cubist picture, Les Demoiselles d’ Avignon, 1906-07). [1]

Several more recent discussions of Cubism have expressed skepticism about the connection between Cubism and relativity. Edward Fry suggests that “while undoubtedly a rough metaphorical parallel may legitimately be made, it would seem dangerous to extend it too far ...;” [2] while both Robert

[Page 412:]

Motherwell, in his edition of The Cubist Painters, and Christopher Gray explain Apollinaire’s “fourth dimension” as a metaphor. [3] In effect, Motherwell and Laporte conduct a debate in their writings over the metaphorical versus the more-than-metaphorical use of this term. Up to this point the question has remained unresolved, but with a closer study of the development and content of Einstein’s Special and General Theories of Relativity and of Minkowski’s space-time continuum and with a reappraisal of the Cubist texts, a conclusive answer may finally be found. The true meaning of the “fourth dimension” and “non-Euclidean geometry” will become clear and the “metaphor debate” will be found unnecessary.

There are four primary passages from Cubist literature which are cited in connection with this subject, and these are quoted in full here so that the reader may examine them. [4] The principal reference is found in the third chapter of Apollinaire’s Les Peintres Cubistes of 1913, which had been given as a lecture in November, 1911 and published previously in the spring of 1912. [5] Apollinaire writes,

The new artists have been violently attacked for their preoccupation with geometry. Yet geometrical figures are the essence of drawing. Geometry, the science of space, its dimensions and relations, has always determined the norms and rules of painting.

Until now, the three dimensions of Euclid’s geometry were sufficient to the restiveness felt by great artists yearning for the infinite.

The new painters do not propose, any more than did their predecessors, to be geometers. But it may be said that geometry is to the plastic arts what grammar is to the art of the writer. Today, scientists no longer limit themselves to the three dimensions of Euclid. The painters have been led quite naturally, one might say by intuition, to preoccupy themselves with the new possibilities of spatial measurement which, in the language of the modern studios, are designated by the term: the fourth dimension.

Regarded from the plastic point of view, the fourth dimension appears to spring from the three known dimensions: it represents the immensity of space eternalizing itself in all directions at any given moment. It is space itself, the dimension of the infinite; the fourth dimension endows objects with plasticity. It gives the object its right proportions on the whole, whereas in Greek art, for instance, a somewhat mechanical rhythm constantly destroys the proportions.

. . . . . . . .

Finally I must point out that the fourth dimension—this Utopian expression should be analyzed and explained, so that nothing more than historical interest may be attached to it—has come to stand for the aspirations and premonitions

[Page 413:]

of the many young artists who contemplate Egyptian, negro, and oceanic sculptures, meditate on various scientific works, and live in anticipation of a sublime art. [6]

The second important passage occurs in Du Cubisme, the essay of 1912 by Gleizes and Metzinger:

This is understood by the Cubist painters, who tirelessly study pictorial form and the space which it engenders.

This space we have negligently confused with pure visual space or with Euclidean space.

Euclid, in one of his postulates, speaks of the indeformability of figures in movement, so we need not insist upon this point.

If we wished to tie the painter’s space to a particular geometry, we should have to refer it to the non-Euclidean scientists; we should have to study, at some length, certain of Riemann’s theorems. [7]

Maurice Raynal mentions the fourth dimension in an essay, “Qu’est-ce que . . . le “Cubisme’?,” which appeared in Cœmedia illustré on December 20, 1913:

Instead of painting objects as they [the Primitives] saw them, they painted them as they thought them, and it is precisely this law that the cubists have re-adopted, amplified and codified under the name of “The Fourth Dimension.”

The cubists, not having the mysticism of the Primitives as a motive for painting, took from their own age a kind of mysticism of logic, of science and reason, and this they have obeyed like the restless spirits and seekers after truth that they are. [8]

Finally, in his introduction to the catalogue of the Cubist exhibition held by the Mánes Society in Prague during February and March of 1914, Alexandre Mercereau writes,

Our artists ardently desire to achieve an integral truth as opposed to an apparent reality. In harmony with the innovations of science, today’s art seeks to discover ultimate laws more profound than those of yesterday. But just as the principles postulated by Bolyai, Lobatschewski, Riemann, Beltrami and de Tilly have not destroyed those of Euclid but merely relegated them to their true status as one postulate among many,... the modern painter does not presume to negate everything accomplished before his time. [9]

* * * * * *

[Page 414:]

Can the expressions “the fourth dimension” and “non-Euclidean geometry” in these writings be construed as references to the relativity of Einstein and the space-time of Minkowski, even though the names of these scientists never appear in Cubist literature? Discussing Apollinaire’s mention of the fourth dimension, Fry has suggested, “One can only conjecture as to where Apollinaire himself got this notion, unless possibly from contemporary popularizations of Einstein’s 1905 special theory of relativity.’’ [10] To answer this question, the content of the Special Theory of Relativity and of Minkowski’s famous lecture of 1908 must be analyzed.

Einstein published his Special Theory of Relativity (which must be distinguished from his General Theory of Relativity, of 1916) in an article, “On the Electrodynamics of Moving Bodies,” in Annalen der Physik of 1905. The basic idea of the theory is that the laws of nature are the same for all systems moving uniformly with respect to each other. Adding that the velocity of light must be a constant, c, Einstein then uses the equations of the Lorentz-Einstein transformation to replace the old addition of velocities and to preserve the invariance of natural laws in a moving system. [11] From these equations it follows that both distance, measured in the direction of motion, and time will be modified as the velocity increases, and thus are relative rather than absolute quantities. Similarly, Einstein posits the relativity of the mass of a moving electron to which relativistic kinetic energy is added. [12]

Where are the fourth dimension and non-Euclidean geometry in the Special Theory of 1905? The answer is, they are nowhere to be found. If “the fourth dimension” might have come from a foreshadowing in 1905 of Minkowski’s integration of time with the three dimensions of space into a four-dimensional space-time continuum, an historian of science stresses a fundamental point:

It is important to understand that at this stage Einstein had discovered only the Lorentz-Einstein transformations together with their inevitable relativistic consequences. There is not the slightest hint, in his writings, of a world of space-time. [13]

Likewise, the search for non-Euclideanism is fruitless. Einstein’s moving systems are “Galilean frames,” which are given by Cartesian co-ordinates with different orientations to signify relative velocities. In marked contrast to the General Theory of Relativity, of 1916, in which Riemann’s non-Euclidean geometry would indeed help Einstein to explain the structure of the space-time continuum, in 1905 “it does not appear that Einstein was influenced in the slightest degree by Riemann’s ideas.’’ [14]

[Page 415:]

Because the concept of simultaneity does play a part in Einstein’s discussion of the relativity of time in 1905, some authors have attempted to draw a parallel with “simultaneity” in Cubist works in which separate points of view are presented at the same time. Thus, Siegfried Giedion has written, “It is a temporal coincidence that Einstein should have begun his famous work (Zur Electrodynamik bewegter Körper) with a careful definition of simultaneity.” [15] What has been overlooked by these authors is Einstein’s purpose, which was to make simultaneity obsolete. His statement in 1905 reads as follows:

So we see that we cannot attach any absolute signification to the concept of simultaneity, but that two events which, viewed from a system of co-ordinates, are simultaneous, can no longer be looked upon as simultaneous events when envisaged from a system which is in motion relatively to that system. [16]

Thus, even had Einstein’s negative view of simultaneity been known to them, it is hardly likely that it would have encouraged Cubist painters to show several views of an object or to bring objects widely separated in space together, as in “epic” Cubism. [17] In fact, the impossibility of relating Cubist techniques to the Special Theory of Relativity was pointed out to Paul Laporte by Einstein himself, who wrote in a letter of 1946, “This new artistic ‘language’ has nothing in common with the Theory of Relativity.’’[18]

On September 21, 1908 Hermann Minkowski gave a lecture entitled “Space and Time” before the 80th Assembly of German Natural Scientists and Physicians at Cologne. He began,

The views of space and time which I wish to lay before you have sprung from the soil of experimental physics, and therein lies their strength. They are radical. Henceforth space by itself, and time by itself, are doomed to fade away into mere shadows, and only a kind of union of the two will preserve an independent reality. [19]

The purpose of Minkowski’s formulation of a four-dimensional continuum with three dimensions of space and one of time was to synthesize the points of view of all observers after Einstein had made them relative in 1905. In the equation dx2+dy2+dz2-c2dt2=ds2 (now an invariant quantity), which was the mathematical representation of Einstein’s premises, Minkowski discovered that he could describe the location of a point-event in a four-dimensional continuum. Using the word “substance” to refer to every participant in the continuum, he proposed individual “world-lines,” whose paths are deter-

[Page 416:]

mined by dx, dy, dz, and dt. [20] This, the space of our Galilean frame at one onstant is a cross-section of the continuum and our time is perpendicular to this section.

In this way the fourth dimension finally appears in scientific writing, but non-Euclidean geometry, its usual companion in art-historical analyses of Cubism, is a crucial omission from Minkowski’s lecture. In discussing Minkowski’s space-time of 1908, Christopher Gray has written, “The new space was to be a four-dimensional Riemannian hyper-space, an isotropic continuum in which the time dimension was always essential in defining relationships.” [21] Similarly, Laporte, in his case for Cubism and the developments in relativity in 1905 and 1908, argues that “the integration of non-Euclidean geometry with the fourth dimension is a constituent factor in contemporary physics.” [22] But if the geometrical nature of Minkowski’s four-dimensional continuum is complex, it certainly cannot be labelled as simply non-Euclidean or Riemannian. Actually, it is quite Euclidean in the sense that its dx2, dy2, and dz2 terms indicate a flat area free from curvature or non-Euclideanism. Minkowski temporarily solved the problem presented by the -c2dt2 term by introducing the √-1 to make the time dimension imaginary, an acceptable alternative within Euclidean geometry. In addition to the fact that Riemann’s name is not once mentioned in Minkowski’s lecture, the final proof of the Euclidean nature of the space-time continuum in 1908 is provided by Einstein himself. Writing in later years of the development of relativity theory, Einstein summarized Minkowski’s great contribution as “. . . his recognition that the four-dimensional space-time continuum of the theory of relativity, in its most essential formal properties, shows a pronounced relationship to the three-dimensional continuum of Euclidean space.” [23]

Indeed, the “four-dimensional Riemannian hyper-space”’ mistakenly attri- buted by Gray to Minkowski in 1908 was only developed later by Einstein and was published for the first time as his General Theory of Relativity in 1916. By 1911 Einstein was convinced of the equivalence of inertial and gravitational mass [24] and, with the impetus of Minkowski’s space-time discovery, sought to incorporate the effect of gravitational fields produced by matter into the space- time continuum. Einstein’s final formulation, published in Annalen der Physik, was much like that of Riemann, who had asserted theoretically that the structure of any metrical field was affected by the forces of matter within that field. [25] The flatness of space-time was thus broken up into pockets of non-Euclideanism in the areas around matter, and Einstein even adopted the Riemann-

[Page 417:]

Christoffel Tensor, using tensor calculus to replace the earlier Lorentz-Einstein transformations. [26] Clearly, the mistake of art historians anxious to explain references to the fourth dimension and non-Euclidean geometry has been to read back into Cubist writings of 1911 and 1912 a breakthrough in physics which was not published until 1916.

* * * * * *

Even if relativity theory as it stood in 1911 and 1912 had been better suited to the Cubist ideals, contemporary popularizations of the theories of Einstein and Minkowski did not exist. Contrary to Fry’s suggestion of such popular knowledge of the Special Theory of 1905 and the assumption by others of a widespread familiarity with Minkowski’s space-time, there was virtually no notice by the general public of these discoveries until 1919. In that year, during an eclipse of the sun, light rays could be seen to be bent by the mass of the sun, as Einstein’s General Theory had predicted. Determining how much these theories were known prior to 1919 is indeed a difficult task, but a number of different factors confirm the unlikelihood that the Cubist painters and writers could have known anything of Special Relativity or of space-time. The popular magazine L’Illustration carried nothing on these topics during the years in question, although a section of each issue was devoted to current scientific discoveries. The complete absence until 1919 of listings for “Einstein,” “Minkowski,” “Relativity,” and “Space-Time” in the Readers’ Guide to Periodical Literature reinforces the notion that the theories were not popularized, particularly since they did not even appear in more scientifically-oriented journals, such as Scientific American and Science.

On a higher scientific level, only in 1912 was a paper given at the American Academy of Arts and Sciences in relation to Minkowski’s lecture of 1908. In “The Space-Time Manifold of Relativity: The Non-Euclidean Geometry of Mechanics and Electro-Magnetics,” Edwin Wilson and Gilbert Lewis of the Massachusetts Institute of Technology were developing a non-Euclidean geometry for space-time for the first time. The presence of this innovation at this level in November, 1912 makes a similar formulation by Cubist writers in 1911-12 about paintings of 1909 through 1912 nearly impossible. The paper by Wilson and Lewis was the source of knowledge of relativity for an Ameri can mathematician, Henry Parker Manning, who mentioned it for the first

[Page 418:]

time in 1914 in his Geometry of Four Dimensions. Although Manning had published several books between 1905 and 1914, only in 1914 does he write (with a footnote to Wilson and Lewis),

Another very important application of geometry of four dimensions is that mentioned by d’Alembert, making time the fourth dimension; within a few years this idea has been developed fully, and has been found to furnish the simplest statement of the new physical principle of relativity. [27]

Finally, the French mathematician and scientist Henri Poincaré has been described as a supporter of Einstein in the early days when his theory was attacked. [28] However, rarely in any of his writings does Poincaré mention Einstein or Minkowski by name. Certainly in the books published close to 1905 and 1908 Poincaré was not an advocate of either of these two scientists.

Another famous figure who was in Paris during those crucial years may serve as an effective gauge of the knowledge of relativity that was current in French intellectual circles. In 1907 Bergson had published L’ Evolution créatrice, in which he had focused on the active elements of time, duration and becoming as the vital ingredients in human existence. Bergson’s flow of time was necessarily absolute, but he sensed no need in 1907 even to mention Einstein’s new relativity of time. Again in 1911 Bergson must not have felt that his ideas were endangered by the theories of Einstein or Minkowski, for in his lecture at Oxford in that year, “La Perception du changement,” time remained absolute and no refutation of Einstein was required. Not until 1922 was Bergson compelled to answer the challenge to his doctrine inherent in the Special Theory of Relativity. In the introduction to Duration and Simultaneity (With Reference to Einstein's Theory), Bergson explains his motive:

We began it solely for our own benefit. We wanted to find out to what extent our conception of duration was compatible with Einstein’s views on time.

. . . . . . . .

We had in the past extended an effort in that direction. The theory of relativity has supplied us with the occasion for resuming it and carrying it a bit further. [29]

Bergson succeeds in his own mind in bringing Einstein’s relativity into line with Bergsonian philosophy by differentiating between the relativity of Einstein and that of Lorentz. According to the author, it is Lorentz, and not Einstein, who posits the existence of the forbidden multiple times, all equally real. He concludes triumphantly, although perhaps not completely soundly,

[Page 419:]

“Far from ruling out the hypothesis of a single, universal duration, Einstein’s theory of special relativity calls for it and confers upon it a superior intelligibility.” [30]

* * * * * *

If we have succeeded in eliminating the possibility of Einstein and Minkowski to explain mentions of the fourth dimension and non-Euclidean geometry as they appear in Cubist literature, what remains to account for the use of these terms? Actually, the answer is quite straightforward, although it has been completely overlooked in the past. Instead of relativity, the concepts enjoying contemporary popularity were simply four-dimensional geometry and, to a lesser extent, non-Euclidean geometry: by 1911 the bibliography of works on the geometry of n dimensions (four or more) comprised 1,832 references, one-third Italian, one-third German, and the rest mainly French, English and Dutch. [31]

The idea of geometries of higher dimensions had developed in the nineteenth century out of both analytic and synthetic geometry. In analytical geometry the step was made naturally, as early as 1833, from equations containing more than three variables. Möbius was the first to suggest that mirror images could be made to coincide if space had four dimensions, and the 1840s and 1850s saw additional writings on the subject by Cayley, Veronese and Sylvester. [32] Mathematical treatises on n-dimensional geometry continued to be produced throughout the nineteenth century, but the fourth dimension soon found its way into popular literature as well. In 1885 E. A. Abbott wrote one of the earliest and most famous examples of this, Flatland: A Romance of Many Dimensions by a Square. The charming story tells of an inhabitant of two-dimensional Flatland, who is granted a vision of Spaceland. Once there with his guide, a Sphere, the Square’s inquiring mind leads him to ask,

But my Lord has shown me the intestines of all my countrymen in the Land of Two Dimensions by taking me into the Land of Three. What therefore more easy than now to take his servant on a second journey into the blessed region of the Fourth Dimension, where I shall look with him once more upon this land of Three Dimensions, and see the inside of every three-dimensional house, the secrets of the solid earth, the treasures of the mines of Spaceland, and the intestines of every solid living creature, even of the noble and adorable Spheres. [33]

The Square’s new-found open-mindedness, however, is not appreciated by

[Page 420:]

his three-dimensional companion and he is hurled back to Flatland and to imprisonment for his tales of the third dimension.

A fourth dimension which might exist but could not be seen by beings of the three-dimensional world continued to fascinate the public during the pre-war years of the twentieth-century. Under “Fourth Dimension,” the Readers’ Guide for that period lists innumerable articles, in publications ranging from Current Literature and McClure’s Magazine to The Popular Science Monthly. Even more indicative of the interest in the fourth dimension at this time is a contest sponsored by Scientific American in 1909, with a prize of $500 “. . . which was to be awarded . . . for the best popular explanation of the Fourth Dimension, the object being to set forth in an essay not longer than twenty-five hundred words the meaning of the term so that the ordinary lay reader could understand it.” [34] The contest generated a great deal of interest, and entries were received from the United States, Turkey, Austria, Holland, India, Australia, France and Germany. Published in a collection in 1910, the best essays serve as another factor weighing against the notion of public knowledge of the theories of Einstein and Minkowski, for no parallels are drawn with four-dimensional space-time. The author of the prize-winning essay was obviously unaware of these discoveries when he speculated that “the limitation of space to three dimensions, though probably correct, [was] purely empirical.” [35]

The Scientific American contest essays are a good measure of current ideas about the fourth dimension. Nearly every author makes certain to disassociate his interest in the geometrical fourth dimension and its possible scientific applications (e.g., as an explanation of certain characteristics of electricity) from the use of the fourth dimension by spiritualists as the home of their specters. The primary challenge to each writer is to visualize and to describe the fourth dimension, an exceedingly difficult task since that dimension must be perpendicular to each of the three known dimensions. Just as a three-dimensional figure is bounded by planes, a four-dimensional figure would be bounded by solids. Such a figure must necessarily be viewed in sections, either by passing it through our space so that new sections continually appear or by turning it on an axis. None of these authors succeeds with the difficult problem much more than had Howard Hinton in his The Fourth Dimension, of 1904, when by means of an elaborate color system he presented the four-dimensional cube or “tesseract,” hardly discernible by anyone other than the author.

Geometry texts had begun to appear in this period which could do a somewhat better job of portraying the fourth dimension with the aid of analytical

[Page 421:]

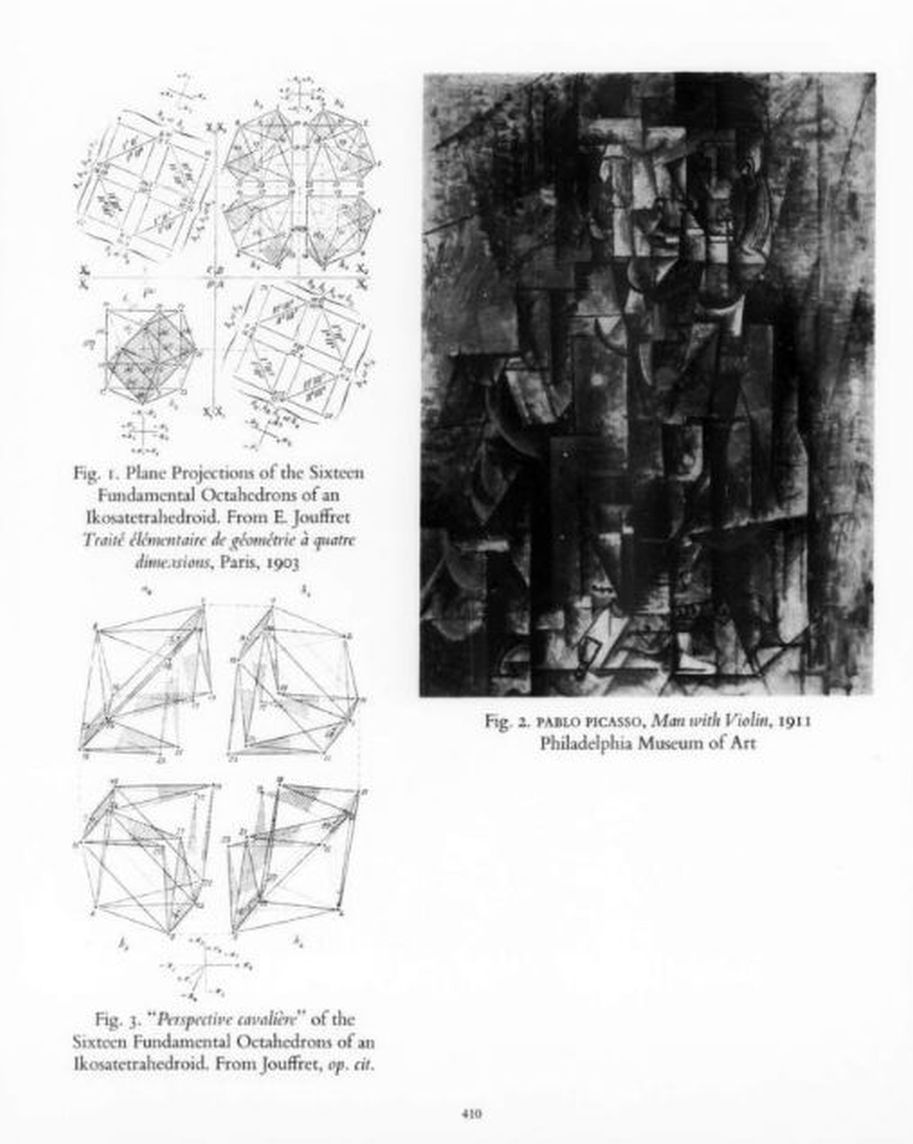
geometry to generate the figures. One such book is E. Jouffret’s Traité élémentaire de géométrie à quatre dimensions, published in Paris in 1903. Jouffret employs “descriptive geometry,” in which figures are developed by analytical geometry and then dissected for projection on a two-dimensional page, continually being turned and juxtaposed. Fig. 1 demonstrates the method for sixteen of the twenty-four octahedrons which make up the four-dimensional ikosatetrahedroid. Nevertheless, Jouffret recognizes the limitations of his success in representing the fourth dimension:

M. Poincaré has said, no doubt ironically: “One who devoted his life to it would perhaps eventually be able to picture the fourth dimension.”

For our part, we have already stated our opinion. It is that the reader should not hope to objectify, as does the blindfolded chess player with the pieces on his mental chessboard, either the four-dimensional beings which are the object of this study, or the movements that we impart to them; he would exhaust his intelligence in vain efforts seeking to break through the plane which extends between those beings and himself. If there are really four dimensions, our mind is confined in the first three. [36]

The representation of the fourth dimension would have been an equally great challenge to the Cubist painters. Their interest in mathematics in the years about 1910 is generally agreed upon. [37] The mysterious Maurice Princet, a friend of Picasso and of other Cubist artists, was an insurance actuary, and his knowledge of mathematics is often said to have influenced the painters. [38] In view of the great popularity of the idea of the fourth dimension at the end of the nineteenth century, Princet most likely had been introduced to the geometry of higher dimensions in the course of his mathematical training for his work as an actuary. Perhaps the most convincing evidence of the artists’ interest in four-dimensional geometry is the comparison of an Analytical Cubist work—for example, Picasso’s Man with Violin (Fig. 2), executed at the end of 1911—with a typical illustration from Jouffret (Fig. 3). Jouffret’s drawing is a “perspective cavalière,” or see-through view, of the four-dimensional body in Fig. 1, showing certain facets of all sixteen octahedrons at one time. The variety of planes seen from various angles and the shifting relationships created by the shading of certain of these planes provide a striking similarity to the Cubist work. With the excitement about the geometry of four dimensions so widespread in this period, it is not surprising that the fourth dimension would have fascinated artists and become a part of the “language of the modern studios,” as Apollinaire has stated.

* * * * * *

[Page 422:]

The references to non-Euclidean geometry and to certain non-Euclidean mathematicians in Gleizes and Metzinger and others may likewise be traced to a current interest in non-Euclidean geometry, much like that in the geometry of n dimensions. In fact, although the curved space of non-Euclideanism is a separate phenomenon from n-dimensional geometry, the two had been joined in the work of Riemann about 1850. Riemann’s positively curved space was elliptic, while the earlier formulations of Bolyai and Lobachevski had been negatively curved and hyperbolic. In France, by the first decade of the twentieth century, non-Euclidean geometry had its own special champion in the person of Henri Poincaré, the mathematician, scientist and writer mentioned previously in connection with the lack of popular knowledge of Einstein and Minkowski. It is no coincidence that the emphasis of Gleizes and Metzinger in their essay of 1912, Du Cubisme, is upon non-Euclidean geometry, for it can be shown that they were relying in part upon Poincaré’s book La Science et l’ Hypothèse, of 1902.

Poincaré’s chapter on “Space and Geometry” would have been of great interest to the Cubist authors in general, for he discusses both “The ‘Non-Euclidean World” and “The World of Four Dimensions.” More specifically, Poincaré’s analysis of the three forms of perceptual space—visual, tactile and motor—appears to be the source for the thoughts of Gleizes and Metzinger on pictorial space. Poincaré begins by contrasting geometric space with the levels of spatial perception created by our sensations. Visual space exists on two levels, “pure visual space” being the two-dimensional image formed on the retina and “complete visual space,” the first level of perception of a third dimension. Poincaré writes of “complete visual space,”

But everyone knows that this perception of the third dimension reduces itself to the sensation of the effort at accommodation it is necessary to make, and to that of the convergence which must be given to the two eyes, to perceive an object distinctly. [39]

Similarly, Gleizes and Metzinger write that “as for visual space, we know that it results from the harmony of the sensations of convergence and accommodation of the eye.’’ [40]

Immediately following this section on visual space, Poincaré continues with his second and third forms of perceptual space, tactile and motor:

TACTILE SPACE AND MOTOR SPACE.—“Tactile space” is still more

[Page 423:]

complicated than visual space and farther removed from geometric space. It is superfluous to repeat for touch the discussion I have given for sight.

But apart from the data of sight and touch, there are other sensations which contribute as much and more than they to the genesis of the notion of space. These are known to every one; they accompany all our movements, and are usually called muscular sensations.

The corresponding frame constitutes what may be called motor space.

Each muscle gives rise to a special sensation capable of augmenting or of diminishing, so that the totality of our muscular sensations will depend upon as many variables as we have muscles. From this point of view, motor space would have as many dimensions as we have muscles. [41]

Poincaré’s last sentence would have been especially intriguing for Gleizes and Metzinger, but his ideas on tactile and motor space were evidently equally valuable to these Cubist authors. One paragraph after their discussion of visual space, Gleizes and Metzinger state, “To establish pictorial space, we must have recourse to tactile and motor sensations, indeed to all our faculties.” [42] Their conclusion that pictorial space must be “a sensitive passage between two subjective spaces’’ [43] seems closely related to Poincaré’s establishment of tactile and motor as highly subjective, perceptual spaces far removed from geometric space.

With this evidence of Gleizes and Metzinger’s reading of Poincaré, their famous statement with its non-Euclidean reference, which was quoted earlier, becomes more comprehensible. Their assertion that pictorial space has negligently been confused with “pure visual space” or with “Euclidean space” is logical in the light of Poincaré’s separation of geometric space from the forms of perceptual space, the least subjective of these being “pure visual space.” The lack of inner consistency in the next two sentences may perhaps be explained as the result of the Cubist authors’ borrowing from Poincaré ideas they did not completely understand. Aside from the fact that Poincaré mentions Euclid’s postulate of the indeformability of figures in movement in the preceding chapter, “The Non-Euclidean Geometries,” it hardly makes sense for Gleizes and Metzinger, who are leading up to an advocacy of non-Euclidean geometry, to state this Euclidean concept. It is most likely that this very chapter of Poincaré, with its elucidation of the geometries of the various “non-Euclidean scientists,” was the source for the study of Riemann’s theorems which Gleizes and Metzinger suggest. In general, the popular books of Poincaré, such as La Science et l’ Hypothèse, may well have been the “scientific works” over which

[Page 424:]

the aspiring young Cubist artists described by Apollinaire were “meditating.”

* * * * * *

Once the connection of the Cubist painters with the geometry of four dimensions and non-Euclidean geometry is established, one may begin to reconstruct the way in which this liaison developed. From the limited amount of evidence available at the present time, a course of events emerges in which Jean Metzinger plays a central role. The first hint of Cubist knowledge of four-dimensional geometry occurs in Metzinger’s “Note sur la peinture,” which appeared in Pan for October-November, 1910:

. . . Picasso brings us a material account of their [forms’] real life in the mind— he lays out a free mobile perspective, from which that ingenious mathematician Maurice Princet has deduced a whole geometry. [44]

Princet’s mathematical training and his probable familiarity with the geometry of four dimensions have already been mentioned. Although Picasso has denied that he ever discussed mathematics or the fourth dimension with Princet, [45] the general popularity of the fourth dimension and the availability of his friend Princet’s ideas, and perhaps even of texts about geometry, make at least an indirect influence on Picasso seem plausible. An interesting speculation by Louis Vauxcelles about the source of Princet’s knowledge of the fourth dimension appears in a letter from Juan Gris to Maurice Raynal in February of 1919. Gris refers to an unspecified issue of Carnet de la Semaine, in which Vauxcelles allegedly stated that “Princet, a clever mathematician, learned about the fourth dimension through reading the works of Poincaré.’’ [46] Gris’s cynical view of the Vauxcelles article is cause to doubt its historical validity, but it is interesting that the name of Poincaré is associated with the Cubist ideals. Although Princet would certainly have had other sources for his mathe- matical knowledge, he may well have been the one to recommend Poincaré to Metzinger and Gleizes.

Gleizes and Metzinger had met by late 1909 or early 1910 and had exhibited together with Léger, Delaunay and Le Fauconnier in Salle 41 of the Salon des Indépendants of 1911. Soon this group of Cubists was in contact with Villon, Duchamp-Villon, La Fresnaye, Duchamp and Picabia, meeting frequently at Villon’s home in Puteaux or at Gleizes’s studio in Courbevoie. The fourth dimension and non-Euclidean geometry were popular topics of discussion among the artists; [47] and to judge from the recollections of Marcel Duchamp,

[Page 425:]

Metzinger seems to have been a major figure when the conversation turned to mathematics. [48] In November of 1911 Apollinaire composed his famous discussion of the fourth dimension which later became the third chapter of Les Peintres Cubistes. 1912 brought the collaboration of Gleizes and Metzinger in writing Du Cubisme with the certain aid of Poincaré’s La Science et l’ Hypothèse. And, finally, in 1913 Metzinger paid a last tribute to the fourth dimension, entitling one of his still lifes, Nature morte (4me dimension). [49]

Marcel Duchamp, himself, provides a most important postscript to this development. In two separate notes for the Large Glass, Duchamp specifically mentions Jouffret and Poincaré by name. The Jouffret reference occurs in a note full of Duchamp’s speculation about the fourth dimension, which can be dated to late 1912 or early 1913. [50] The artist begins, “The shadow cast by a 4-dimensional figure on our space is a 3-dimensional shadow (see—Jouffret Geom. a 4 dim. page 186. last 3 lines).” [51] This notion, so essential to Duchamp’s conception of the Bride, was indeed a popular one and may well have emerged during the discussions at Puteaux and Courbevoie. Duchamp cites Poincaré in one of the notes published in the White Box. [52] Certainly Duchamp’s interest in the non-Euclidean geometry associated with Poincaré is evidenced in the Three Standard Stoppages of 1913-14. Whether or not Duchamp went beyond his fellow artists and actually read Lobachevski and Riemann, as has been claimed, [53] his relationship to current ideas about the fourth dimension and non-Euclidean geometry should be studied further.

* * * * * *

It is worthwhile to examine exactly what the concepts “geometry”, “non-Euclidean geometry” and “the fourth dimension” meant to the Cubists in terms of their painting. In the literature of Cubism, simple “geometry” has the connotation of old-fashioned Euclidean geometry and lacks all the glamor of the fourth dimension and non-Euclidean geometry. From the first, observers of Cubist paintings had noticed a similarity with well-known geometrical solids and had been critical of this—as Apollinaire states, “The new artists have been violently attacked for their preoccupation with geometry.” Apollinaire, however, is sympathetic to the idea that simple geometry may have some worth; he writes, “Geometry, the science of space, its dimensions and relations, has always determined the norms and rules of painting.” But Gleizes

[Page 426:]

and Metzinger, on their part, join in the diatribe against the outmoded geometry of Euclid:

Geometry is a science, painting is an art, The geometer measures, the painter savors. The absolute of one is necessarily the relative of the other; . . .

We are frankly amused to think that many a novice may perhaps pay for his too literal comprehension of Cubist theory, and his faith in absolute truth, by arduously juxtaposing the six faces of a cube or the two ears of a model seen in profile. [54]

Clearly, in the minds of Gleizes and Metzinger their involvement in the modern non-Euclidean and four-dimensional geometries entitles them to a status far above that of “geometer,” the label they constantly deny.

As advanced as the Cubists may have thought themselves, however, there is some confusion in their understanding of the difference between non-Euclidean geometry and the geometry of n dimensions, a confusion shared by many laymen at that time. In the sense that Euclid never dealt with more than three dimensions, four-dimensional geometry might be thought of as non-Euclidean, even though true non-Euclidean geometry (normally of three or less dimensions) is defined by its rejection of certain of Euclid’s postulates. That Apollinaire thinks of four-dimensional geometry as non-Euclidean is demonstrated when he introduces the fourth dimension by explaining, “Today, scientists no longer limit themselves to the three dimensions of Euclid.” At the same time, it is likely that Gleizes and Metzinger interpret their non-Euclidean geometry as a geometry of higher dimensions. Thus, they relate their pictorial space to a non-Euclidean geometry which, unlike “pure visual space” or “Euclidean space,” includes Poincaré’s tactile and motor space possessing “. . . as many dimensions as we have muscles.” [55]

There seems to be more general agreement among the Cubist authors on the way in which the fourth dimension aids the modern painter. Apollinaire writes,

[The fourth dimension] is space itself, the dimension of the infinite; the fourth dimension endows objects with plasticity. It gives the object its right proportions on the whole, whereas in Greek art, for instance, a somewhat mechanical rhythm constantly destroys the proportions.

For Apollinaire, the fourth dimension is also the means of overcoming perspective, “that miserable tricky perspective,” “that fourth dimension in reverse,” that infallible device for making all things shrink,” as he terms it. [56]

[Page 427:]

Associating perspective with the highly undesirable Euclidean geometry, Gleizes and Metzinger would surely have concurred with Apollinaire’s opposition of the fourth dimension to perspective.

Gleizes and Metzinger also echo Apollinaire’s idea of the fourth dimension as the space in which the correct proportions of an object are to be found:

The worth of a river, foliage, and banks, despite a conscientious faithfulness to scale, is no longer measured by width, thickness, and height, nor the relations between these dimensions. Torn from natural space, they have entered a different kind of space, which does not assimilate the proportion observed. [57]

The popular notion of the fourth dimension as the true reality of which we can see only three dimensions would have justified the freedom from observed reality which Gleizes and Metzinger claim. It is as if the quest for the unseen fourth dimension entitles the artist to adjust at will the proportions, scale and arrangement of the elements in his painting, guided only by the way he visualizes that added dimensionality. Indeed, this conceptual orientation so basic to Cubism was explained in terms of the fourth dimension by Maurice Raynal in 1913:

Instead of painting objects as they [the Primitives] saw them, they painted them as they thought them, and it is precisely this law that the cubists have re- adopted, amplified and codified under the name of “The Fourth Dimension.” [58]

* * * * * *

Two final aspects of the traditional art-historical analysis of Cubism remain to be brought into line with the new interpretation of the fourth dimension and non-Euclidean geometry. These are “space-time” and “‘simultaneity,” both frequently invoked in connection with the relativistic Cubism of Einstein and Minkowski.

The word “space-time” does not come from Cubist literature, but is rather a later application of Minkowski’s terminology to the element of time sensed from the beginning by observers of Cubist works. Roger Allard wrote in 1910 that a Cubist painting offered its viewer “. . . the elements of a synthesis situated in the passage of time.” [59] Metzinger explained this more fully in an article, “Cubisme et Tradition,” which appeared in Paris-Journal in August of 1911:

[The Cubists] have allowed themselves to move round the object, in order to give, under the control of intelligence, a concrete representation of it, made up

[Page 428:]

of several successive aspects. Formerly a picture took possession of space, now it reigns also in time. [60]

Thus, time is the means by which the artist views his object. This must be kept in mind when considering Cubism’s fourth dimension, for, in addition to the geometrical fourth dimension of the Cubists, there is an alternate tradition of time itself as the fourth dimension. As early as the eighteenth century time had been interpreted as a fourth dimension by d’Alembert and Lagrange. [61] This more philosophical fourth dimension, continued in the writings of Howard Hinton, P. D. Ouspensky and Maurice Maeterlinck, is symbolized by the generation of a four-dimensional tesseract simply by moving a cube through time. [62] It is extremely important to distinguish this tradition from the idea of the fourth dimension as a higher geometric reality which beings of a three-dimensional world cannot see. This latter fourth dimension is the one which enjoyed great popularity at the end of the nineteenth century and in the early years of the twentieth. It is the fourth dimension of E. A. Abbott and Flatland, of the Scientific American essays, of Jouffret, of Princet, of Poincaré and of Cubism. Time plays the same supporting role for a Cubist painter seeking the fourth dimension that it does in four-dimensional geometry: it allows either the moving around the object or the turning of the object itself which is necessary to form an idea of its total dimensionality.

The time implied in a Cubist painting is related to the famous concept of “simultaneity”’ as well. [63] In fact, it is through four-dimensional geometry that the nature of Cubist simultaneity is best understood. As they write in Du Cubisme of the day when Cubism will be appreciated, Gleizes and Metzinger describe the method Metzinger had explained earlier in “Cubisme et Tradition:”

Then the fact of moving around an object to seize from it several successive appearances, which, fused into a single image, reconstitute it in time, will no longer make reasoning people indignant. [64]

This is exactly the procedure for representing four-dimensional bodies suggested by Poincaré in La Science et l’ Hypothèse, Poincaré states,

... Just as the perspective of a three-dimensional figure can be made on a plane, we can make that of a four-dimensional figure on a picture of three (or of two) dimensions . . . We can even take of the same figure several perspectives from several different points of view.

. . . . . . . .

In this sense we may say the fourth dimension is imaginable. [65]

[Page 429:]

And, as the perfect illustration of Poincaré’s system (and of that of Gleizes and Metzinger), a textbook of four-dimensional geometry like Jouffret’s presents the various views of an object simultaneously. Indeed, many Analytical Cubist works appear to be the result not of the artist’s simple movement about his object, but of the kind of mental picture of the process of rotation and the resulting interrelationships of facets which the geometer must employ in dealing with the unseen fourth dimension.

* * * * * *

There can be little doubt that the references to the fourth dimension and non-Euclidean geometry in the literature of Cubism are reflections of the early twentieth-century interest in new and more complex forms of geometry. The strong visual parallels which exist between Analytical Cubist paintings and geometrical drawings of four-dimensional figures are reinforced both by the presence among the Cubists of Princet and by Duchamp’s quotation from the Traité élémentaire de géométrie à quatre dimensions of Jouffret. Likewise, Duchamp’s mention of Poincaré supports the proof furnished by Gleizes and Metzinger’s dependence upon Poincaré for their ideas on space in Du Cubisme. Certainly the appeal to the Cubists of Poincaré and his geometries is logical in artistic terms. His approach has a practical, visual quality to it, far more useful for a painter than the abstruse theorizing of Einstein or Minkowski, which was once thought somehow to be the scientific equivalent of Cubism. With the new understanding of the fourth dimension and non-Euclidean geometry, a valuable tool for critical analysis is gained and the art historian is freed from a long-standing dilemma. No longer must he cushion the term “the fourth dimension” by labelling it a mere metaphor or stretch his imagination to see the Cubists discussing the latest developments in relativity theory.

YALE UNIVERSITY

This study originated in a seminar conducted by Professor Robert L. Herbert at Yale University in the Spring of 1970. A shorter version of this paper, “The Location of Cubism’s Fourth Dimension,” was presented as part of a session on “Art, Science and Technology” at the College Art Association convention in January, 1971. I am especially grateful to Professor Herbert for his guidance throughout the course of my study. I am also indebted to Professor Martin J. Klein of the Yale History of Science Department, who generously served as my consultant on the scientific matters dealt with in this text, and to Professor Anne Coffin Hanson, whose comments and suggestions were of great help.

1 Paul M. Laporte, “Cubism and Science,” Journal of Aesthetics and Art Criticism, VII/3, March 1949, p. 254.

One of the earliest discussions of Cubism in terms of relativity is found in Siegfried Giedion’s Space, Time and Architecture of 1939, where he mentions Cubism’s ability to express the unification of space and time. In

[Page 430:]

1942 Walter F. Isaacs applied a similar interpretation to painting in “Time and the Fourth Dimension in Painting,” in the College Art Journal, II/1, November 1942, pp. 2-7. An interesting rebuttal to the Isaacs article was published in 1943 by Joachim Weyl. Refusing to believe the ability of any Cubist to visualize the elusive concept of space-time, Weyl insists that the connection must instead be made with the science of Descartes and Newton. (Joachim Weyl, “Science and Abstract Art,” College Art Journal, II/2, January 1943, pp. 42-46.) Weyl’s argument, however, did not stem the tide of enthusiasm for a “relativistic” Cubism. Laporte’s articles appeared in the late 1940s, and in 1961 Werner Haftmann, in Painting in the Twentieth Century, still presented a timetable of events similar to that of Laporte: “Dates seem to suggest that some kind of connection exists between science and painting. The radical changes in painting took place between 1900 and 1910. Significant dates are: 1905 Fauvism; 1907 Cubism; 1910 the first abstract painting. A concordance of dates important in the history of science runs thus: 1900 Planck’s quantum theory and Freud’s Interpretation of Dreams; 1905 Einstein’s special theory of relativity; 1908 Minkowski’s mathematical formulation of the dimensions of space-time.” (Werner Haftmann, Painting in the Twentieth Century, II, New York, 1965, p. 8.)

2 Edward F. Fry, Cubism, New York, 1966, note, pp. 119-120.

3 See Robert Motherwell, ed., The Cubist Painters (Aesthetic Meditations) (1913), by Guillaume Apollinaire, trans. Lionel Abel, New York, 1949, note 1, p. 51. See also Christopher Gray, Cubist Aesthetic Theories, Baltimore, 1961, note 22, p. 63.

4 These four texts are mentioned in this connection by Edward Fry in his anthology of Cubist writings: Fry, Cubism, p. 32. When phrases or sentences from the excerpts of Apollinaire’s Les Peintres Cubistes and Gleizes and Metzinger’s Du Cubisme recur in the course of the article, they will not be footnoted again and the reader may simply refer back to the initial quotations.

5 Fry, Cubism, note, p. 119.

6 “On a vivement reproché aux artistes-peintres nouveaux des préoccupations géométriques. Cependant les figures géométriques sont l’essentiel du dessin. La géométrie, science qui a pour objet l’étendue, sa mesure et ses rapports, a été de tous temps la régle méme de la peinture.

Jusqu’a présent, les trois dimensions de la géométrie euclidienne suffisaient aux inquiétudes que le sentiment de l’infini met dans l’âme des grands artistes.

Les nouveaux peintres, pas plus que leurs anciens ne se sont proposés d’étre des géométres. Mais on peut dire que la géométrie est aux arts plastiques ce que la grammaire est à l’art de l’écrivain. Or, aujourd’hui, les savants ne s’en tiennent plus aux trois dimensions de la géométrie euclidienne. Les peintres ont été amenés tout naturellement et, pour ainsi dire, par intuition, à se préoccuper de nouvelles mesures possibles de l’étendue que dans le langage des ateliers modernes on désignait toutes ensemble et brièvement par le terme de quatrième dimension.

Telle qu’elle s’offre à l’esprit, du point de vue plastique, la quatriéme dimension serait engendrée par les trois mesures connues: elle figure l’immensité de l’espace s’éternisant dans toutes les directions à un moment déterminé. Elle est l’espace méme, la dimension de l’infini; c’est elle qui doue de plasticité les objets. Elle leur donne les proportions qu’ils méritent dans l’ceuvre, tandis que dans l’art grec par exemple, un rythme en quelque sorte mécanique détruit sans cesse les proportions.

. . . . . . . .

Ajoutons que cette imagination: la quatriéme dimension, n’a été que la manifestation des aspirations, des inqui¢tudes d’un grand nombre de jeunes artistes regardant les sculptures égyptiennes, nègres et océaniennes, méditant les ouvrages de science, attendant un art sublime, et, qu’on n’attache plus aujourd’hui à cette expression utopique, qu’il fallait noter et expliquer, qu’un intérét en quelque sorte historique.” (Guillaume Apollinaire, Les Peintres Cubistes [Méditations Esthétiques] [1913], Geneva, 1950, pp. 17-19.) In English, The Cubist Painters (Aesthetic Meditations) (1913), ed. Robert Motherwell, trans. Lionel Abel, New York, 1949, pp. 13-14.

7 “Les peintres cubistes le savent, qui étudient inlassablement la forme picturale et l’espace qu'elle engendre.

Euclide, en l’un de ses postulats, pose l’indéformabilité des figures en mouvement, cela nous épargne d’insister.

Si l’on désirait rattacher l’espace des peintres à quelque géométrie, il faudrait en référer aux savants non euclidiens, méditer longuement certains théorèmes de Riemann.” (Albert Gleizes and Jean Metzinger, Du Cubisme, Paris, 1912, p. 17.) In English, Cubism, in Modern Artists on Art, ed. Robert L. Herbert, Englewood Cliffs, N.J., 1964, pp. 7-8.

[Page 431:]

8 Maurice Raynal, “Qu’est-ce que . . . le ʻCubisme’?,” Comœdia illustré, Paris, December 20, 1913, in Cubism, ed. Edward Fry, pp. 129-130.

9 Alexandre Mercereau, “Introduction to the catalogue of the forty-fifth exhibition of the Mánes Society, Prague, February-March 1914,” in Cubism, ed. Edward Fry, p. 134.

10 Fry, Cubism, note, p. 119.

11 Lincoln Barnett, The Universe and Dr. Einstein, New York, 1957, p. 55. The equations give the measurements for the moving system as follows:

12 A. d’Abro, The Evolution of Scientific Thought from Newton to Einstein, New York, 1950, p. 157.

13 d’Abro, Evolution of Scientific Thought, p. 432.

14 d’Abro, Evolution of Scientific Thought, p. 458.

15 Siegfried Giedion, Space, Time and Architecture, 3rd ed., Cambridge, Mass., 1965, p. 432.

16 Albert Einstein, “On the Electrodynamics of Moving Bodies” (1905), in The Principle of Relativity, ed. A. Sommerfeld, trans. W. Perrett and G. B. Jeffrey, London, 1923, p. 43.

17 See Fry, Cubism, p. 32. The term “epic” Cubism originated with Daniel Robbins in his article, “From Symbolism to Cubism: The Abbaye of Créteil,” The Art Journal, XXIII/2, Winter 1963-1964, pp. 111-116.

18 Albert Einstein, as quoted in Paul M. Laporte, “Cubism and Relativity,” The Art Journal, XXV/3, Spring 1966, p. 246. In this article Laporte confesses that Einstein did reject the ideas published in his 1948 ond 1949 essays when he submitted them to the scientist several years earlier. Einstein replied in a letter of May 4, 1946, “I find your comparison rather unsatisfactory ... [T]he essence of the Theory of Relativity has been incorrectly understood in it, granted that this error is suggested by the attempts at popularization of the theory.” According to Einstein, the Theory of Relativity says only that general laws do not depend on the system of co-ordinates chosen, not that a number of systems are needed for the representation of a single case, as Laporte had interpreted it. He continues in the letter, “It is completely sufficient to describe the whole mathematically in relation to one system of co-ordinates. This is quite different in the case of Picasso’s painting, as I do not have to elaborate any further. . . . This new artistic ‘language’ has nothing in common with the Theory of Relativity.”

Despite Einstein’s refutation, Laporte argues in the 1966 article that a “. . . ‘correct’ understanding, not only in science but also in art, is possible but to a relatively small number of specialists . . .” and continues to insist that there is indeed a “common denominator for art and science.”

19 Hermann Minkowski, “Space and Time” (1908), in The Principle of Relativity, p. 75.

20 Minkowski, “Space and Time,” p. 76.

21 Gray, Cubist Aesthetic Theories, p. 94.

22 Laporte, “Cubism and Science,” p. 254.

23 Albert Einstein, Relativity: The Special and the General Theory (1916), New York, 1961, p. 57.

24 d’Abro, Evolution of Scientific Thought, p. 436.

25 d’Abro, Evolution of Scientific Thought, p. 458.

26 Albert Einstein, “The Foundation of the General Theory of Relativity,” in The Principle of Relativity, p. 140.

27 Henry Parker Manning, Geometry of Four Dimensions, New York, 1914, p. 11.

28 d’Abro, Evolution of Scientific Thought, p. xvi.

29 Henri Bergson, Duration and Simultaneity (With Reference to Einstein’s Theory) (1922), trans. Leon Jacobson, New York, 1965, pp. 5-6.

30 Bergson, Duration and Simultaneity, p. viii.

31 Manning, Geometry, p. 9.

32 Manning, Geometry, p. 5.

[Page 432:]

33 E. A. Abbott, Flatland: A Romance of Many Dimensions by a Square, Boston, 1885, p. 135.

34 Henry Parker Manning, ed., The Fourth Dimension Simply Explained (A Collection of Essays Selected from Those Submitted in the Scientific American Prize Competition), New York, 1910, p. 3.

35 Lt. Col. Graham Denby Fitch, “A Euclidation of the Fourth Dimension,” in The Fourth Dimension Simply Explained, p. 51. The italics are mine.

36 “M. Poincaré a dit, ironiquement sans doute: ‘Quelqu’un qui y consacrerait son existence, pourrait peut-être arriver à se représenter la quatrième dimension.’ [Revue générale des Sciences, 1891, p. 774.—Cette boutade est expliquée et commentée par M. Poincaré dans une publication ultérieure: L’ Espace et la Géométrie (Revue de Métaphysique et de Morale, pp. 631-646, 1895).]

Pour nous, nous avons déjà dit notre pensée. Elle est que le lecteur ne doit pas caresser l’espoir d’objectiver, comme le blindfold-player le fait avec les pièces de son échiquier mental, les êtres à quatre dimensions qui font l’objet de cette étude, ni les mouvements que nous leur imprimerons; qu’il épuiserait son cerveau dans de stériles efforts en cherchant à percer la tranche infinitésimale qui s’étend entre ces êtres et lui. S’il y a ré-ellement quatre dimensions, notre esprit est confiné dans les trois premières.” (E. Jouffret, Traité élémentaire de géométrie à quatre dimensions, Paris, 1903, p. xvi.)

37 See, for instance, Gray, Cubist Aesthetic Theories, p. 71.

38 Fry, Cubism, note, p. 61. André Warnod, Alice Halicka, and Fernande Olivier have made such assertions. Kahnweiler, however, refused to grant that Princet had an important effect on the Cubists, as does Fry in discussing these opinions.

39 “Mais chacun sait que cette perception de la troisième dimension se réduit au sentiment de l’effort d’accommodation qu’il faut faire, et à celui de la convergence qu'il faut donner aux deux yeux, pour percevoir un objet distinctement.” (Henri Poincaré, La Science et l’ Hypothése, Paris, 1902, pp. 70-71.) In English, in Henri Poincaré, The Foundations of Science: Science and Hypothesis, The Value of Science, and Science and Method, trans. George B. Halsted, New York, 1913, p. 67.

40 “‘Quant à l’espace visuel, on sait qu’il résulte de l’accord des sensations de convergence et d’accommodation.” (Gleizes and Metzinger, Du Cubisme, p. 17.) In English, in Herbert, Modern Artists on Art, p. 8.

41 “L’espace tactile et l’espace moteur.—‘L’ espace tactile’ est plus compliqué encore que l’espace visuel et s’éloigne davantage de l’espace géométrique. II est inutile de répéter, pour le toucher, la discussion que j’ai faite pour la vue.

Mais en dehors des données de la vue et du toucher, il y a d’autres sensations qui contribuent autant et plus qu’elles à la genèse de la notion d’espace. Ce sont celles que tout le monde connait, qui accompagnent tous nos mouvements et que l’on appelle ordinairement musculaires.

Le cadre correspondant constitue ce que l’on peut appeler l’espace moteur.

Chaque muscle donne naissance à une sensation spéciale susceptible d’augimenter ou de diminuer, de sorte que l’ensemble de nos sensations musculaires dépendra d’autant de variables que nous avons de muscles. A ce point de vue, l’espace moteur aurait autant de dimensions que nous avons de muscles.” (Poincaré, La Science et l’Hypothèse, pp. 72-73.) In English, in The Foundations of Science, pp. 68-69.

42 “Pour établir l’espace pictural, il faut recourir à des sensations tactiles et motrices et à toutes nos facultés.” (Gleizes and Metzinger, Du Cubisme, p. 18.) In English, in Herbert, Modern Artists on Art, p. 8.

43 Gleizes and Metzinger, Cubism, in Herbert, Modern Artists on Art, p. 8.

44 Jean Metzinger, “Note sur la peinture,” Pan, Paris, October-November 1910, in Cubism, ed. Edward Fry, p. 60.

45 Alfred H. Barr, Jr., Picasso: Fifty Years of His Art, New York, 1946, p. 259. Barr received this answer from Picasso in a questionnaire of October, 1945.

46 Louis Vauxcelles, as quoted by Juan Gris, Letter to Maurice Raynal, February 15, 1919, in Letters of Juan Gris, ed. and trans. Douglas Cooper, London, 1956, p. 62.

47 See Marcel Duchamp, in “Eleven Europeans in America,” The Museum of Modern Art Bulletin, XIII/4-5, 1946, p. 21. See also William A. Camfield, “La Section d’Or” and Daniel Robbins, “The Genealogy of the Section d’Or,” in Leonard Hutton Galleries, New York, Albert Gleizes and the Section d’Or, October 28- December §, 1964.

48 Duchamp, in “Eleven Europeans in America,” p. 21.

49 Fry, Cubism, p. 112.

50 Arturo Schwarz, ed., Notes and Projects for the Large Glass, trans. George H. Hamilton, Cleve Gray, and Arturo Schwarz, London, 1969, p. 5.

51 Marcel Duchamp, Notes and Projects for the Large Glass, note 3, p. 36.

[Page 433:]

52 This information was given by Mr. K. G. Pontus Hultén in a letter of April 14, 1971. The author has not yet had the opportunity of examining one of the 150 copies of “A l’Infinitif” (The White Box) published by Cordier & Ekstrom in 1967.

53 See, for example, William S. Rubin, Dada and Surrealist Art, New York, 1969, p. 38.

54 Gleizes and Metzinger, Cubism, in Herbert, Modern Artists on Art, p. 13.

55 Poincaré, Science and Hypothesis, in The Foundations of Science, p. 69. See note 41, above.

56 Apollinaire, The Cubist Painters, p. 45.

57 Gleizes and Metzinger, Cubism, in Herbert, Modern Artists on Art, p. 7.

58 Raynal, “Qu’est-ce que . . . le ‘Cubisme’?,” in Cubism, ed. Edward Fry, pp. 129-130.

59 Roger Allard, “Au Salon d’Automne de Paris,” L’Art Libre, Lyons, November 1910, in Cubism, ed. Edward Fry, p. 62.

60 Jean Metzinger, ““Cubisme et Tradition,” Paris-Journal, August 16, 1911, in Cubism, ed. Edward Fry, pp. 66-67.

61 Manning, Geometry, p. 4. D’Alembert published this idea in his article of 1754 on “Dimension” in the Encyclopédie edited by Diderot and himself. Lagrange presented this view in 1797 in his Théorie des fonctions analytiques.

62 See Howard Hinton, The Fourth Dimension, London and New York, 1904; P. D. Ouspensky, Tertium Organum (1911), New York, 1922; and Maurice Maeterlinck, The Life of Space, London, 1928.

63 The word “simultaneity” was not used by the Cubists to describe their juxtaposition of several views of an object until after the term had appeared in the preface to the Bernheim-Jeune Exhibition of the Futurists in February of 1912. (See Marianne Martin, Futurist Art and Theory, 1909-1915, Oxford, 1968, p. 205.) For the Futurists, as for the earlier proto-Cubist Abbaye de Créteil group, “simultaneity” stood for the pace of modern life with its speed and “simultaneity of states of mind.” (See Pär Bergman, “Modernolatrià” et “Simultaneita,” Uppsala, 1962.) By 1914 in Paris the word was associated with the simultaneous contrasts of color of Robert Delaunay. The fact that “simultaneity” was accepted by the painters and theorists after February, 1912 as a description of the Cubist method makes it a more legitimate concept than that of “space-time.” Nevertheless, like “space-time,” “‘simultaneity” was certainly popularized in art-historical writing by the proponents of a connection between Cubism and relativity.

64 “Alors le fait de se mouvoir autour d’un objet pour en saisir plusieurs apparences successives qui, fondues en une seule image, le reconstituent dans la durée, n’indignera plus les raisonneurs.”” (Gleizes and Metzinger, Du Cubisme, p. 36.) In English, in Herbert, Modern Artists on Art, p. 15.

65 “. .. De même qu’on peut faire sur un plan la perspective d’une figure à trois dimensions, on peut faire celle d’une figure à quatre dimensions sur un tableau à trois (ou à deux) dimensions . . .On peut même prendre d’une même figure plusieurs perspectives de plusieurs points de vues différents.

. . . . . . . .

C’est dans ce sens qu’il est permis de dire qu’on pourrait se représenter la quatrième dimension.” (Poincaré, La Science et l’Hypothèse, pp. 89-90.) In English, in The Foundations of Science, pp. 78-79.

SOURCE: Henderson, Linda Dalrymple. “A New Facet of Cubism: ‘The Fourth Dimension’ and ‘Non-Euclidean Geometry’ Reinterpreted,” The Art Quarterly (Winter 1971), pp. 410 (illus.), 411-433.

Martin Gardner, Mathematical Games, & the Fourth Dimension
(web guide & bibliography)

Surrealism: Selected Links

Book Review: Barnett's 'Universe'

Philosophy and the Physicists
by L. Susan Stebbing